Sunil Natarajan
Credit Analyst
Hi David,
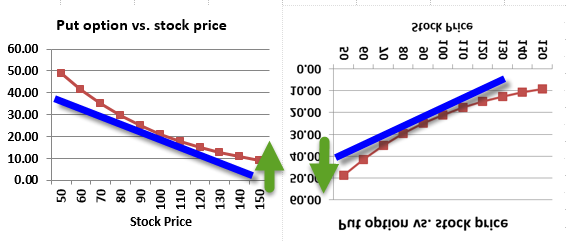
In FRM Handbook Ch-13, Phillipe Jorion has mentioned that bonds always have positive convexity.In options the convexity(Gamma) can be both positive and negative. Long position in an option has positive Gamma, while short position in an option has negative gamma.
I wanted to know whether bonds irrespective of long or short position always have positive convexity. I thought that long position in a bond would have positive convexity and vice versa. And also a call option in a bond creates a negative convexity for a bond holder and a put option on a bond does create a negative convexity position for an issuer.
In FRM Handbook Ch-13, Phillipe Jorion has mentioned that bonds always have positive convexity.In options the convexity(Gamma) can be both positive and negative. Long position in an option has positive Gamma, while short position in an option has negative gamma.
I wanted to know whether bonds irrespective of long or short position always have positive convexity. I thought that long position in a bond would have positive convexity and vice versa. And also a call option in a bond creates a negative convexity for a bond holder and a put option on a bond does create a negative convexity position for an issuer.