Hi,
I have a doubt about the meaning of the hedge ratio.
Hedge ratio = ρ * σ_spot / σ_fut
Number of contracts = HedgeRatio * PortfolioValue / ValueFuturesContract
Therefore, the lower the correlation, the lower the number of contracts.
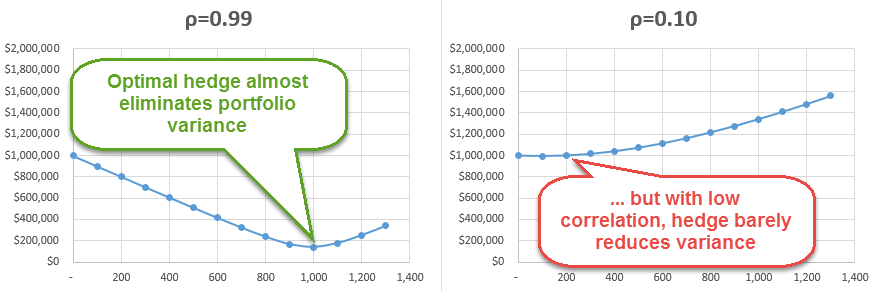
So, let's say that I have a portfolio of $ 1.000.000 of Crude Oil (σ_spot = 100) that I want to hedge. I have two products that I can use to hedge it:
- 1. Crude Oil futures:
- 2. Weather futures:
If I calculate the number of contracts to hedge the position for each product I have:
1. N=0.99*100/100*1.000.000/1.000=990
2. N=0.10*100/100*1.000.000/1.000 = 100
How is it possible that with less correlation the number of contracts will be less? Would it cost less to hedge the position with a less correlated product? It is counterintuitive for me. What am I missing?
Thank you in advance.
I have a doubt about the meaning of the hedge ratio.
Hedge ratio = ρ * σ_spot / σ_fut
Number of contracts = HedgeRatio * PortfolioValue / ValueFuturesContract
Therefore, the lower the correlation, the lower the number of contracts.
So, let's say that I have a portfolio of $ 1.000.000 of Crude Oil (σ_spot = 100) that I want to hedge. I have two products that I can use to hedge it:
- 1. Crude Oil futures:
ρ = 0.99
σ_fut = 100
Value Futures Contract = $ 1.000
σ_fut = 100
Value Futures Contract = $ 1.000
ρ = 0.10
σ_fut = 100
Value Futures Contract = $ 1.000
σ_fut = 100
Value Futures Contract = $ 1.000
If I calculate the number of contracts to hedge the position for each product I have:
1. N=0.99*100/100*1.000.000/1.000=990
2. N=0.10*100/100*1.000.000/1.000 = 100
How is it possible that with less correlation the number of contracts will be less? Would it cost less to hedge the position with a less correlated product? It is counterintuitive for me. What am I missing?
Thank you in advance.