Learning objectives: Explain the arbitrage pricing theory (APT), describe its assumptions and compare the APT to the CAPM. Describe the inputs (including factor betas) to a multifactor model. Calculate the expected return of an asset using a single-factor and a multifactor model. Explain models that account for correlations between asset returns in a multi-asset portfolio. Explain how to construct a portfolio to hedge exposure to multiple factors. Describe and apply the Fama-French three-factor model in estimating asset returns.
Questions:
20.10.1. Consider the following three portfolios where we know the expected excess returns of Portfolios A and B; i.e., E[ER(A)] = 6.0% and E[ER(B)] = 8.4% and these in excess of the riskfree rate. For Portfolios A and B, we also know the factor betas: β(A,1) = 0.40, β(A,2) = 1.20, β(B,1) = 0.80, β(A,1) = 1.50. The unknowns are the two risk premiums on the two factors, F(1) and F(2):
a. 5.00%
b. 7.50%
c. 10.00%
d. Not enough information
20.10.2. Assume the following three-factor arbitrate priding theory (APT) model of the expected rate of return (EROR) for a particular stock, where the three macro factors are gross domestic product (GDP), the inflation rate (IR), and a generic third factor (F3):
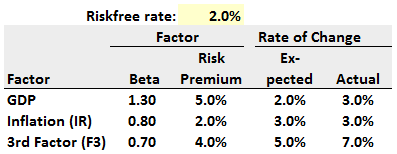
The riskfree rate is 2.0%. The exhibit also shows each factor's risk premium (e.g., the GDP factor's risk premium is 5.0%), its Expected rate of change, and its Actual rate of change. The stock's INITIAL expected rate of return (EROR) assumes that each factor's expected rate of change; e.g., inflation's expected rate of change is 3.0%. The stock's REVISED expected rate of return (EROR) incorporates any surprise (ie.., difference between Actual and Expected rate of change) in the macro factor. Assume we are estimating the stock's gross return (i.e., including the risk-free rate) not its excess return. In regard to the stock's gross expected rate of return (EROR), what is, respectively, the INITIAL EROR and the REVISED EROR?
a. 7.30% and 9.40%
b. 9.10% and 11.80%
c. 12.90% and 15.60%
d. 15.50% and 17.70%
20.10.3. Each of the following statements is true about the arbitrage pricing theory (APT) model EXCEPT which is false?
a. APT says that systemic factors explain asset returns and that diversification both eliminates specific risk and precludes arbitrage opportunities
b. If the return process for 30 firms is a five-factor market model, then the number of parameters to be estimated is 160 parameters as given by 30*5 + 5*(5-1)/2
c. As a special case of the capital asset pricing model, a key advantage of the APT is that matrix transformation identifies via deductive guidance the model's relevant macroeconomic factors and their risk premiums
d. The extended 2015 Fama-French model includes five factors, the market's price of risk, small minus big (SMB), high minus low (HML), robust minus weak (RMW) and conservative minus aggressive (CMA), but they found HLM and CMA somewhat redundant
Answers:
Questions:
20.10.1. Consider the following three portfolios where we know the expected excess returns of Portfolios A and B; i.e., E[ER(A)] = 6.0% and E[ER(B)] = 8.4% and these in excess of the riskfree rate. For Portfolios A and B, we also know the factor betas: β(A,1) = 0.40, β(A,2) = 1.20, β(B,1) = 0.80, β(A,1) = 1.50. The unknowns are the two risk premiums on the two factors, F(1) and F(2):
- Portfolio A: E[ER(A)] = β(A,1)*F(1) + β(A,2)*F(2) = 0.40*F(1) + 1.20*F(2) = 6.0%
- Portfolio B: E[ER(B)] = β(B,1)*F(1) + β(B,2)*F(2) = 0.80*F(1) + 1.50*F(2) = 8.4%
- Portfolio C has the following betas: β(C,1) = 1.30 and β(C,2) = 0.90
a. 5.00%
b. 7.50%
c. 10.00%
d. Not enough information
20.10.2. Assume the following three-factor arbitrate priding theory (APT) model of the expected rate of return (EROR) for a particular stock, where the three macro factors are gross domestic product (GDP), the inflation rate (IR), and a generic third factor (F3):

The riskfree rate is 2.0%. The exhibit also shows each factor's risk premium (e.g., the GDP factor's risk premium is 5.0%), its Expected rate of change, and its Actual rate of change. The stock's INITIAL expected rate of return (EROR) assumes that each factor's expected rate of change; e.g., inflation's expected rate of change is 3.0%. The stock's REVISED expected rate of return (EROR) incorporates any surprise (ie.., difference between Actual and Expected rate of change) in the macro factor. Assume we are estimating the stock's gross return (i.e., including the risk-free rate) not its excess return. In regard to the stock's gross expected rate of return (EROR), what is, respectively, the INITIAL EROR and the REVISED EROR?
a. 7.30% and 9.40%
b. 9.10% and 11.80%
c. 12.90% and 15.60%
d. 15.50% and 17.70%
20.10.3. Each of the following statements is true about the arbitrage pricing theory (APT) model EXCEPT which is false?
a. APT says that systemic factors explain asset returns and that diversification both eliminates specific risk and precludes arbitrage opportunities
b. If the return process for 30 firms is a five-factor market model, then the number of parameters to be estimated is 160 parameters as given by 30*5 + 5*(5-1)/2
c. As a special case of the capital asset pricing model, a key advantage of the APT is that matrix transformation identifies via deductive guidance the model's relevant macroeconomic factors and their risk premiums
d. The extended 2015 Fama-French model includes five factors, the market's price of risk, small minus big (SMB), high minus low (HML), robust minus weak (RMW) and conservative minus aggressive (CMA), but they found HLM and CMA somewhat redundant
Answers:
