Learning objectives: Interpret the forward rate, and compute forward rates given spot rates. Define par rate and describe the equation for the par rate of a bond. Interpret the relationship between spot, forward, and par rates.
Questions:
903.1. Assume the following discount function (note that a discount function is a series of discount factors):
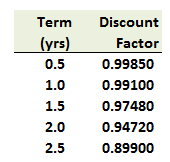
Which of the following is nearest to the implied six-month forward rate starting in two years, f(2.0, 2.5)? Assume rates are expressed as per annum with semi-annual compounding.
a. 4.30%
b. 5.83%
c. 7.95%
d. 10.72%
903.2. Assume the following discount function:

Which of the following is nearest to the 2.5-year par rate (aka, par yield), denoted by Tuckman as C(2.5)? Assume rates are expressed as per annum with semi-annual compounding.
a. 4.40%
b. 4.47%
c. 6.00%
d. 6.18%
903.3. In regard to the relationship between spot, forward and par rates, each of the following statements is necessarily true EXCEPT which is inaccurate? Assume rates are expressed as per annum with semi-annual compounding.
a. If the spot rate curve is strictly upward-sloping, then par rates are below equal-maturity spot rates
b. If the spot rate curve is strictly downward-sloping, then par rates are above equal-maturity spot rates
c. If the 2.5 year spot rate, s(2.5) = 1.40%, and the 3.0 year spot rate, s(3.0) = 1.50%, then the six-month forward rate starting in 2.5 years, f(2.5, 3.0), must be either less than 1.40% or greater than 1.50%
d. The three-year spot rate, r(3.0), is an approximation of the simple average of six of the following six-month forward rates: f(0, 0.5), f(0.5, 1.0), f(1.0, 1.5), f(1.5, 2.0), f(2.0, 2.5), f(2.5, 3.0) where f(0.5) equals s(0.5)
Answers here:
Questions:
903.1. Assume the following discount function (note that a discount function is a series of discount factors):

Which of the following is nearest to the implied six-month forward rate starting in two years, f(2.0, 2.5)? Assume rates are expressed as per annum with semi-annual compounding.
a. 4.30%
b. 5.83%
c. 7.95%
d. 10.72%
903.2. Assume the following discount function:

Which of the following is nearest to the 2.5-year par rate (aka, par yield), denoted by Tuckman as C(2.5)? Assume rates are expressed as per annum with semi-annual compounding.
a. 4.40%
b. 4.47%
c. 6.00%
d. 6.18%
903.3. In regard to the relationship between spot, forward and par rates, each of the following statements is necessarily true EXCEPT which is inaccurate? Assume rates are expressed as per annum with semi-annual compounding.
a. If the spot rate curve is strictly upward-sloping, then par rates are below equal-maturity spot rates
b. If the spot rate curve is strictly downward-sloping, then par rates are above equal-maturity spot rates
c. If the 2.5 year spot rate, s(2.5) = 1.40%, and the 3.0 year spot rate, s(3.0) = 1.50%, then the six-month forward rate starting in 2.5 years, f(2.5, 3.0), must be either less than 1.40% or greater than 1.50%
d. The three-year spot rate, r(3.0), is an approximation of the simple average of six of the following six-month forward rates: f(0, 0.5), f(0.5, 1.0), f(1.0, 1.5), f(1.5, 2.0), f(2.0, 2.5), f(2.5, 3.0) where f(0.5) equals s(0.5)
Answers here:
