Concept: These on-line quiz questions are not specifically linked to learning objectives, but are instead based on recent sample questions. The difficulty level is a notch, or two notches, easier than bionicturtle.com's typical question such that the intended difficulty level is nearer to an actual exam question. As these represent "easier than our usual" practice questions, they are well-suited to online simulation.
Questions:
702.1. You want to estimate the 99.0% expected shortfall (ES) but it is much easier to compute the extreme quantiles of the non-normal distribution, which are shown below:
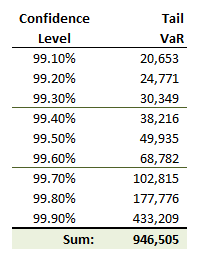
Which is nearest to a reasonable estimate of the 99.0% expected shortfall (ES) of this distribution?
a. $20,653
b. $49,935
c. $94,650
d. $105,176
702.2. What is the primary advantage of a bootstrap historical simulation in comparison to basic (aka, simple) historical simulation?
a. Bootstrap natively and naturally incorporates time dependency
b. Bootstrap allows for easier construction of confidence interval around VaR or ES
c. Bootstrap applies non-parametric density estimation to achieve a smoother distribution
d. Bootstrap is not constrained by largest loss in the historical dataset
702.3. You have collected 100 days (K = 100) of portfolio returns and sorted them. The worst six returns are displayed below. In addition to the returns, you compared the weights implied by simple historical simulation (i.e., 1/100 = 1.0%, 2/100 = 2.0%, ...) to the weights implied by an age-weighted historical simulation (aka, "hybrid" approach) where the lambda, λ, parameter is set to 0.90.

As a technical note, rather than view the worst loss observation (i.e., 5.30%) as the quantile for 1.00% probability (in the simple HS approach) or the 2.29% probability (in the age-weighted HS approach), you are going to view each loss observation as a random variable with a probability mass centered on the observation. In this way, for example, under the simple HS the 99.0% VaR would be 4.75% = average(5.30%, 4.20%) rather than 5.30.
Which of the following is nearest to the 95.0% confident age-weighted HS?
a. 2.50%
b. 2.70%
c. 3.10%
d. 3.70%
Answers here:
Questions:
702.1. You want to estimate the 99.0% expected shortfall (ES) but it is much easier to compute the extreme quantiles of the non-normal distribution, which are shown below:

Which is nearest to a reasonable estimate of the 99.0% expected shortfall (ES) of this distribution?
a. $20,653
b. $49,935
c. $94,650
d. $105,176
702.2. What is the primary advantage of a bootstrap historical simulation in comparison to basic (aka, simple) historical simulation?
a. Bootstrap natively and naturally incorporates time dependency
b. Bootstrap allows for easier construction of confidence interval around VaR or ES
c. Bootstrap applies non-parametric density estimation to achieve a smoother distribution
d. Bootstrap is not constrained by largest loss in the historical dataset
702.3. You have collected 100 days (K = 100) of portfolio returns and sorted them. The worst six returns are displayed below. In addition to the returns, you compared the weights implied by simple historical simulation (i.e., 1/100 = 1.0%, 2/100 = 2.0%, ...) to the weights implied by an age-weighted historical simulation (aka, "hybrid" approach) where the lambda, λ, parameter is set to 0.90.

As a technical note, rather than view the worst loss observation (i.e., 5.30%) as the quantile for 1.00% probability (in the simple HS approach) or the 2.29% probability (in the age-weighted HS approach), you are going to view each loss observation as a random variable with a probability mass centered on the observation. In this way, for example, under the simple HS the 99.0% VaR would be 4.75% = average(5.30%, 4.20%) rather than 5.30.
Which of the following is nearest to the 95.0% confident age-weighted HS?
a. 2.50%
b. 2.70%
c. 3.10%
d. 3.70%
Answers here:
