Learning objectives: Estimate VaR using a historical simulation approach. Estimate VaR using a parametric approach for both normal and lognormal return distributions.
Questions:
707.1. A mutual fund's daily returns for the last 300 trading days is plotted on this histogram. Additionally, the worst 20 daily returns are sorted explicitly below the histogram:
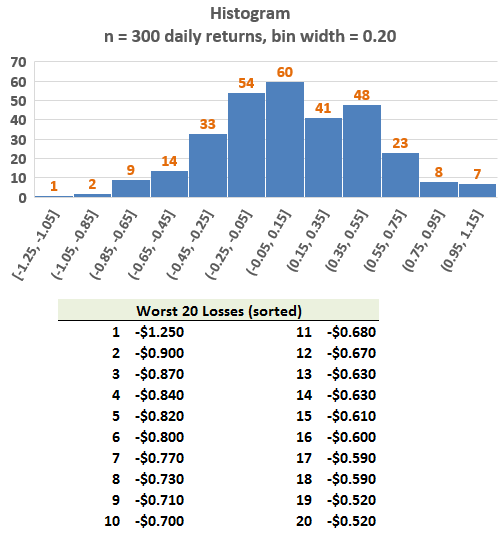
Under the basic historical simulation approach, which of the following is the BEST one-day 99.0% value at risk (VaR)?
a. 0.710
b. 0.840
c. 0.800
d. 0.900
707.2. Consider the following asset over three periods that starts with an initial price of $20.00 and pays a $2.00 dividend each period. In addition to the asset's price and dividends over three periods, we also show its per-period arithmetic (aka, simple) return:
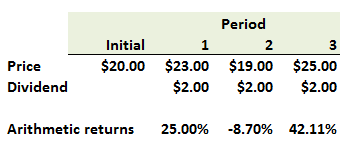
About this asset's performance, each of the following statements is true EXCEPT which is not accurate?
a. Geometric returns cannot be greater than arithmetic returns
b. The geometric returns in each period are approximately +22.31%, -9.10% and 35.14%
c. If the asset instead paid zero dividends, then the geometric return would equal the arithmetic return
d. The three-period geometric return is conveniently the sum of per-period geometric returns which, in this case, is about +22.31% -9.10% +35.14% = 48.36%
707.3. A risk manager is estimating the market risk of a portfolio using both the normal distribution and the lognormal distribution assumptions. The manager gathers the following data on the portfolio:
a. Lognormal 95% VaR is greater than than normal 95% VaR at the one-day holding period by about 2.43%
b. Lognormal 95% VaR is less than than normal 95% VaR at the one-year (250 days) holding period by about 3.90%
c. Lognormal 99% VaR is greater than normal 99% VaR at the one-day holding period by about 4.04%
d. Lognormal 99% VaR is less than normal 99% VaR at the one-year (252 days) holding period by about 21.75%
Answers here:
Questions:
707.1. A mutual fund's daily returns for the last 300 trading days is plotted on this histogram. Additionally, the worst 20 daily returns are sorted explicitly below the histogram:

Under the basic historical simulation approach, which of the following is the BEST one-day 99.0% value at risk (VaR)?
a. 0.710
b. 0.840
c. 0.800
d. 0.900
707.2. Consider the following asset over three periods that starts with an initial price of $20.00 and pays a $2.00 dividend each period. In addition to the asset's price and dividends over three periods, we also show its per-period arithmetic (aka, simple) return:

About this asset's performance, each of the following statements is true EXCEPT which is not accurate?
a. Geometric returns cannot be greater than arithmetic returns
b. The geometric returns in each period are approximately +22.31%, -9.10% and 35.14%
c. If the asset instead paid zero dividends, then the geometric return would equal the arithmetic return
d. The three-period geometric return is conveniently the sum of per-period geometric returns which, in this case, is about +22.31% -9.10% +35.14% = 48.36%
707.3. A risk manager is estimating the market risk of a portfolio using both the normal distribution and the lognormal distribution assumptions. The manager gathers the following data on the portfolio:
- Annual mean: 12.0%
- Annual volatility: 37.0%
- Current portfolio value: USD $1.1 million due to 12,500 shares at a current price of $80.00 per share
- Trading days in a year: 250
a. Lognormal 95% VaR is greater than than normal 95% VaR at the one-day holding period by about 2.43%
b. Lognormal 95% VaR is less than than normal 95% VaR at the one-year (250 days) holding period by about 3.90%
c. Lognormal 99% VaR is greater than normal 99% VaR at the one-day holding period by about 4.04%
d. Lognormal 99% VaR is less than normal 99% VaR at the one-year (252 days) holding period by about 21.75%
Answers here:
Last edited by a moderator:
