Learning Objectives: Describe the significance of estimating default correlation for credit portfolios and distinguish between reduced form and structural default correlation models. Describe the Gaussian copula model for time to default and calculate the probability of default using the one-factor Gaussian copula model. Describe how to estimate credit VaR using the Gaussian copula and the CreditMetrics approach.
Questions:
24.21.1. A risk manager at a regional bank has a diversified loan portfolio. The local economy is facing a downturn due to the declining mining industry. Within the portfolio, two exposures stand out:
Which model is most suitable, and why?
a. A Reduced Form Model assuming hazard rates for different companies follow stochastic processes correlated with macroeconomic variables.
b. A Structural Model based on the value of the company's assets relative to a threshold, with default correlation introduced by assuming correlated stochastic processes for the assets of Alpha Inc. and Delta Inc.
c. A Single-factor Gaussian Copula Model using a single common factor to model likelihood of default, simplifying the correlation structure.
d. A Multivariate Regression Model employing multiple economic indicators to predict default probabilities.
24.21.2. You are a risk analyst tasked with simulating default scenarios for a portfolio of 10 companies over the next 5 years using the Gaussian copula model. The cumulative probabilities of default for each company over the next 1, 2, 3, 4, and 5 years are set at 1%, 3%, 6%, 10%, and 15%, respectively, with a default correlation of 0.2 between each pair of companies.
Using the Gaussian copula model, how would you determine if a default occurs within the first year for any company?
a. Simulate a value from a multivariate normal distribution for each company. If the value is less than the inverse cumulative normal distribution value at 1% (-2.33), default occurs within the first year.
b. Calculate the cumulative probability for each company independently without using a copula. If the probability is less than 1%, assume default occurs within the first year.
c. Use a single-factor model to compute default probability without transforming the values into a normal distribution. Default is assumed if the factor exceeds the threshold set for the first year.
d. Sum the individual default probabilities; if the total exceeds 10%, conclude that a default occurs within the portfolio's first year.
24.21.3. You are tasked with estimating the Credit Value at Risk (Credit VaR) for a significant portfolio that includes two companies:
Given these thresholds and the correlation, how would you implement the Gaussian copula and CreditMetrics to simulate credit rating changes and calculate the Credit VaR?
a. Simulate the credit rating changes for each company independently, ignoring the correlation and common market factors.
b. Utilize the Gaussian copula model to estimate correlated default times based on the provided correlation and threshold values. Use CreditMetrics to simulate credit rating changes for the AAA and BBB companies using the provided thresholds and calculate Credit VaR through Monte Carlo simulations.
c. Assume a fixed default probability for all companies in the portfolio and compute Credit VaR without considering the detailed transition matrix and correlations.
d. Compute Credit VaR by treating both companies as having the same default time and recovery rate, simplifying the model to ignore variations in credit risk profiles.
Answers here:
Questions:
24.21.1. A risk manager at a regional bank has a diversified loan portfolio. The local economy is facing a downturn due to the declining mining industry. Within the portfolio, two exposures stand out:
- Alpha Inc.: A supplier of machinery to the mining industry.
- Delta Inc.: A logistics provider specializing in transportation for mining operations.
Which model is most suitable, and why?
a. A Reduced Form Model assuming hazard rates for different companies follow stochastic processes correlated with macroeconomic variables.
b. A Structural Model based on the value of the company's assets relative to a threshold, with default correlation introduced by assuming correlated stochastic processes for the assets of Alpha Inc. and Delta Inc.
c. A Single-factor Gaussian Copula Model using a single common factor to model likelihood of default, simplifying the correlation structure.
d. A Multivariate Regression Model employing multiple economic indicators to predict default probabilities.
24.21.2. You are a risk analyst tasked with simulating default scenarios for a portfolio of 10 companies over the next 5 years using the Gaussian copula model. The cumulative probabilities of default for each company over the next 1, 2, 3, 4, and 5 years are set at 1%, 3%, 6%, 10%, and 15%, respectively, with a default correlation of 0.2 between each pair of companies.
Using the Gaussian copula model, how would you determine if a default occurs within the first year for any company?
a. Simulate a value from a multivariate normal distribution for each company. If the value is less than the inverse cumulative normal distribution value at 1% (-2.33), default occurs within the first year.
b. Calculate the cumulative probability for each company independently without using a copula. If the probability is less than 1%, assume default occurs within the first year.
c. Use a single-factor model to compute default probability without transforming the values into a normal distribution. Default is assumed if the factor exceeds the threshold set for the first year.
d. Sum the individual default probabilities; if the total exceeds 10%, conclude that a default occurs within the portfolio's first year.
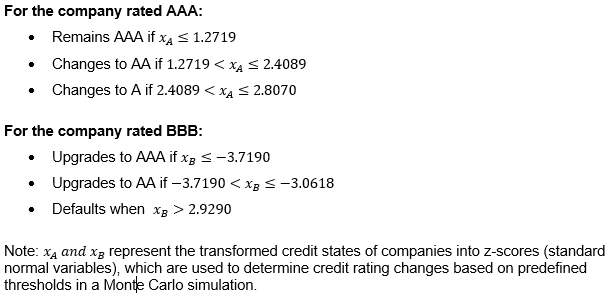
24.21.3. You are tasked with estimating the Credit Value at Risk (Credit VaR) for a significant portfolio that includes two companies:
- The first company was rated AAA
- The second company was rated BBB

Given these thresholds and the correlation, how would you implement the Gaussian copula and CreditMetrics to simulate credit rating changes and calculate the Credit VaR?
a. Simulate the credit rating changes for each company independently, ignoring the correlation and common market factors.
b. Utilize the Gaussian copula model to estimate correlated default times based on the provided correlation and threshold values. Use CreditMetrics to simulate credit rating changes for the AAA and BBB companies using the provided thresholds and calculate Credit VaR through Monte Carlo simulations.
c. Assume a fixed default probability for all companies in the portfolio and compute Credit VaR without considering the detailed transition matrix and correlations.
d. Compute Credit VaR by treating both companies as having the same default time and recovery rate, simplifying the model to ignore variations in credit risk profiles.
Answers here:
