Fran
Administrator
AIMs: Explain how default risk for a single company can be modeled as a Bernoulli trial. Explain the relationship between exponential and Poisson distributions. Define the hazard rate and use it to define probability functions for default time and conditional default probabilities.
Questions:
307.1. Peter the municipal bond analyst observes that in recent years there have occurred only about 6.0 U.S. municipal defaults per year. If he makes the highly simplifying assumption that 6.0 defaults per year is the average in a Poisson process (distribution), what is the probability that the next municipal default will occur within one month?
a. 8.42%
b. 17.00%
c. 39.35%
d. 60.65%
307.2. Suppose the hazard rate (aka, default intensity and denoted by lambda) is constant and equal to 0.090. In this case, each of the following is true EXCEPT which is false?
a. The unconditional one-year default probability is ~ 8.6%
b. The unconditional two-year (aka, cumulative) default probability is ~ 16.5%
c. The probability of joint event of survival through the first year and default in the second year is ~ 7.9%
d. The conditional one-year default probability, given survival through the first year, is ~ 17.3%
307.3. Malz gives us the following two distributions which employ the hazard rate (λ, lambda). Also, as a reminder, the chain rule is shown applied to the exponential function; specifically, exp(x) is elegantly its own derivative, but the derivative of exp[g(x)] is exp[g(x)]*d[g(x)]/dx:
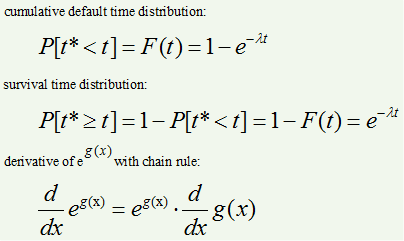
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
Given these default time and survival time distribution functions, each of the following statements is true EXCEPT which is false?
a. As (t) grows large, the cumulative survival time, 1 - F(t), converges to zero such that even a highly-rated company (i.e., low hazard rate) will eventually default
b. Over a very short interval (t, t + Δ) the conditional default probability is given by F(t)/exp(-λ*t)
c. The marginal default probability, which is derivative of the cumulative default time distribution, is given by F'(t) = λ*exp(-λ*t)
d. The marginal survival probability, which is derivative of the cumulative survival time distribution, is given by -F'(t) = -λ*exp(-λ*t) < 0
Answers:
Questions:
307.1. Peter the municipal bond analyst observes that in recent years there have occurred only about 6.0 U.S. municipal defaults per year. If he makes the highly simplifying assumption that 6.0 defaults per year is the average in a Poisson process (distribution), what is the probability that the next municipal default will occur within one month?
a. 8.42%
b. 17.00%
c. 39.35%
d. 60.65%
307.2. Suppose the hazard rate (aka, default intensity and denoted by lambda) is constant and equal to 0.090. In this case, each of the following is true EXCEPT which is false?
a. The unconditional one-year default probability is ~ 8.6%
b. The unconditional two-year (aka, cumulative) default probability is ~ 16.5%
c. The probability of joint event of survival through the first year and default in the second year is ~ 7.9%
d. The conditional one-year default probability, given survival through the first year, is ~ 17.3%
307.3. Malz gives us the following two distributions which employ the hazard rate (λ, lambda). Also, as a reminder, the chain rule is shown applied to the exponential function; specifically, exp(x) is elegantly its own derivative, but the derivative of exp[g(x)] is exp[g(x)]*d[g(x)]/dx:
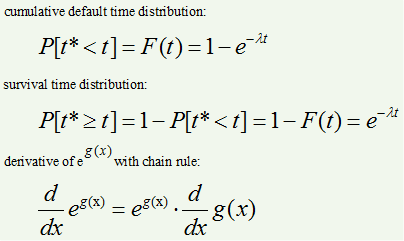
(Source: Allan Malz, Financial Risk Management: Models, History, and Institutions (Hoboken, NJ: John Wiley & Sons, 2011))
Given these default time and survival time distribution functions, each of the following statements is true EXCEPT which is false?
a. As (t) grows large, the cumulative survival time, 1 - F(t), converges to zero such that even a highly-rated company (i.e., low hazard rate) will eventually default
b. Over a very short interval (t, t + Δ) the conditional default probability is given by F(t)/exp(-λ*t)
c. The marginal default probability, which is derivative of the cumulative default time distribution, is given by F'(t) = λ*exp(-λ*t)
d. The marginal survival probability, which is derivative of the cumulative survival time distribution, is given by -F'(t) = -λ*exp(-λ*t) < 0
Answers:

