Learning objectives: Describe a stress test that can be performed on CVA. Calculate the stressed CVA and the stress loss on CVA. Calculate the debt value adjustment (DVA) and explain how stressing DVA enters into aggregating stress tests of CCR. Describe the common pitfalls in stress testing CCR.
Questions:
708.1. Thomas is your firm's Counterparty Credit Risk (CCR) Manager and he is analyzing the credit risk of a portfolio that consists of two sub-portfolios: a traditional pool of funded loans plus a sub-portfolio consisting of unfunded but collateralized credit derivative positions. For each traditional loan, he calculates the expected loss (EL) as the product of its probability of default, p(i), exposure at default, EAD(i), and loss given default, LGD(i). Consequently, expected loss for the sub-pool of (n) traditional loans is given by:
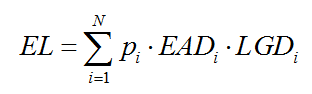
In addition to estimating expected loss for the traditional loan pool and the credit derivative portfolio, Thomas wants to estimated the "stress loss" for the entire portfolio where the "stress loss," SL, is the difference between the portfolio's stressed expected loss, EL(s), and its expected loss; i.e., SL = EL(s) - EL. This exercise will require developing an expected loss and a stressed expected loss for the credit derivatives sub-portfolio, in order that expected losses and stressed expected losses can be summed across the two sub-portfolios. If he begins with the traditional expected loss (EL) calculation above and modifies it in order to produce an CCR-type version of the stressed EL, EL(s) for the derivatives sub-portfolio, then he is likely to make each of the following modifications to the formula EXCEPT which is probably NOT appropriate?
a. For both sub-portfolios, he can retain default probability, p(i), and stress it to higher stressed default probability, p(i)^s
b. For the derivatives sub-portfolio, replace EAD(i) with alpha multiplied by expected positive exposure, α*EPE(i)
c. For the derivatives sub-portfolio, stress exposure at default EAD(i) to higher stressed exposure at default, EAD(i)^s
d. For the derivatives sub-portfolio, stress expected positive exposure, EPE(i), to higher stressed expected positive exposure, EPE(i)^s
708.2. The formula below is given by Lynch as the simplified formula for credit value adjustment (CVA):
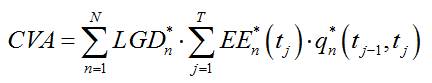
If this CVA formula is applied to a single derivative instrument with a five (5) year life, and the interval between dates, q_n[t(j-1), t(j)], is three months, then each of the following statements is true EXCEPT which is false?
a. Stressed CVA is likely to shock EE(n) to the stressed expected exposure, EE(n)^s
b. To capture wrong-way risk, the formula should add a debt value adjustment (DVA) term
c. Stressed CVA is likely to shock q(n) to the stressed risk-neutral marginal default probability, q(n)^s
d. The expected exposure and default probability will be estimated at twenty (20) points in time
708.3. The formula below is given by Lynch as the bilateral credit value adjustment (bilateral CVA; although the typo is corrected where he means to include NEE rather than duplicate EE):
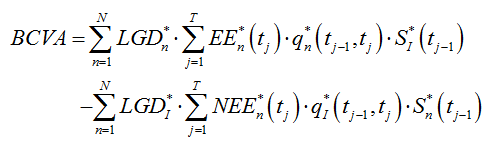
Acme Bank is applying this BCVA formula in regard to measuring credit counterparty exposure to its counterparty; and, further, Acme will stress test this BCVA. Each of the following statements is true EXCEPT which is false?
a. If Acme's own credit quality improves, Acme experiences a loss on the BCVA
b. For stress testing this BCVA, subjective expected exposures should be replaced by observable current exposures
c. Compared to unilateral CVA, BCVA adds S(I) and S(n) which refer to survival probabilities of Acme, S(n), and its counterparty, S(I)
d. BCVA allows CCR to be treated as a market risk, which means CCR can be incorporated in to market risk stress testing in a coherent and additive manner
Answers here:
Questions:
708.1. Thomas is your firm's Counterparty Credit Risk (CCR) Manager and he is analyzing the credit risk of a portfolio that consists of two sub-portfolios: a traditional pool of funded loans plus a sub-portfolio consisting of unfunded but collateralized credit derivative positions. For each traditional loan, he calculates the expected loss (EL) as the product of its probability of default, p(i), exposure at default, EAD(i), and loss given default, LGD(i). Consequently, expected loss for the sub-pool of (n) traditional loans is given by:
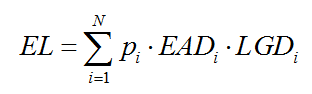
In addition to estimating expected loss for the traditional loan pool and the credit derivative portfolio, Thomas wants to estimated the "stress loss" for the entire portfolio where the "stress loss," SL, is the difference between the portfolio's stressed expected loss, EL(s), and its expected loss; i.e., SL = EL(s) - EL. This exercise will require developing an expected loss and a stressed expected loss for the credit derivatives sub-portfolio, in order that expected losses and stressed expected losses can be summed across the two sub-portfolios. If he begins with the traditional expected loss (EL) calculation above and modifies it in order to produce an CCR-type version of the stressed EL, EL(s) for the derivatives sub-portfolio, then he is likely to make each of the following modifications to the formula EXCEPT which is probably NOT appropriate?
a. For both sub-portfolios, he can retain default probability, p(i), and stress it to higher stressed default probability, p(i)^s
b. For the derivatives sub-portfolio, replace EAD(i) with alpha multiplied by expected positive exposure, α*EPE(i)
c. For the derivatives sub-portfolio, stress exposure at default EAD(i) to higher stressed exposure at default, EAD(i)^s
d. For the derivatives sub-portfolio, stress expected positive exposure, EPE(i), to higher stressed expected positive exposure, EPE(i)^s
708.2. The formula below is given by Lynch as the simplified formula for credit value adjustment (CVA):
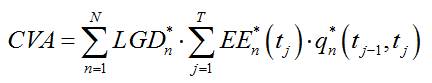
If this CVA formula is applied to a single derivative instrument with a five (5) year life, and the interval between dates, q_n[t(j-1), t(j)], is three months, then each of the following statements is true EXCEPT which is false?
a. Stressed CVA is likely to shock EE(n) to the stressed expected exposure, EE(n)^s
b. To capture wrong-way risk, the formula should add a debt value adjustment (DVA) term
c. Stressed CVA is likely to shock q(n) to the stressed risk-neutral marginal default probability, q(n)^s
d. The expected exposure and default probability will be estimated at twenty (20) points in time
708.3. The formula below is given by Lynch as the bilateral credit value adjustment (bilateral CVA; although the typo is corrected where he means to include NEE rather than duplicate EE):
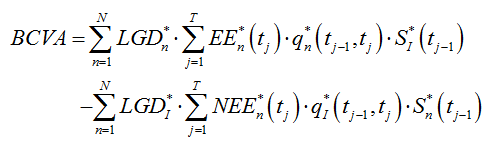
Acme Bank is applying this BCVA formula in regard to measuring credit counterparty exposure to its counterparty; and, further, Acme will stress test this BCVA. Each of the following statements is true EXCEPT which is false?
a. If Acme's own credit quality improves, Acme experiences a loss on the BCVA
b. For stress testing this BCVA, subjective expected exposures should be replaced by observable current exposures
c. Compared to unilateral CVA, BCVA adds S(I) and S(n) which refer to survival probabilities of Acme, S(n), and its counterparty, S(I)
d. BCVA allows CCR to be treated as a market risk, which means CCR can be incorporated in to market risk stress testing in a coherent and additive manner
Answers here:
Last edited by a moderator:
