Question on Swaps Hull chapter 7

[SP_SK]

Hi David,

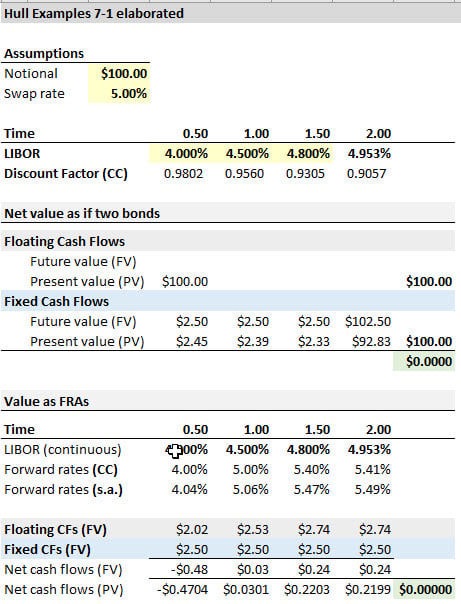
while going through the video and slides, I had a question the LOS regarding explain "Explain how the discount rates in a plain vanilla interest rate swap are computed.". in the two examples, 9th and 10th edition, why does the first boot strap of the libor spot rate compute to a final PV of 100 and then solve for z4 but the 10th edition example, that uses OIS discount rate, do a difference in the swap and libor forward rate and solve for z4 post computing a final PV of 0. could we have done the 10th edition example as only taking coupons discounted by OIS and solved for a PV of 100 and then calculated z4? please explain. Thanks.

 

[David Harper CFA FRM]

Hi @SP_SK Yes, that is possibly confusing. I copied each below. In the 9th edition, he is bootstrapping to infer a 2-year zero rate (notice the "R") but in the 10th edition, emphasis mine, notice he provides the 2-year (OIS) zero rate and goes on to solve for the 2-year forward rate (notice solving for "F").

This is a sub-class of an overall theme in Hull's 10th edition. In the 9th edition (and previously), LIBOR was typically (or implicit) the riskfree discount rate. However, Hull has written for a long time, going back to the crises, about how LIBOR is not the best riskfree rate. In the 10th edition, he formalized the switch:

From Hull's Preface: What’s New in the Tenth Edition?

"Material has been updated and improved. OIS discounting is now used throughout the book. This makes the presentation of the material more straightforward and more theoretically appealing. The valuation of instruments such as swaps and forward rate agreements requires (a) forward rates for the rate used to calculate payments (usually LIBOR) and (b) the risk-free zero curve used for discounting (usually the OIS zero curve). The methods presented can be extended to situations where payments are dependent on any risky rate."-- Hull, John C.. Options, Futures, and Other Derivatives (Page xx). Pearson Education. Kindle Edition.

The example 7.2 (10th edition) below is part of that; it sets up the valuation of an interest rate swap where the floating cash flows are based on a (slightly) different rate (LIBOR) than the discounting rate. I hope that explains!

Example 7.1:

Suppose that the 6-month, 12-month, and 18-month LIBOR/swap zero rates have been determined as 4%, 4.5%, and 4.8% with continuous compounding and that the 2-year swap rate (for a swap where payments are made semiannually) is 5%. This 5% swap rate means that a bond with a principal of $100 and a semiannual coupon of 5% per annum sells for par. It follows that, if R is the 2-year zero rate, then

2.5*exp(-0.04*0.5) + 2.5*exp(-0.045*1.0) + 2.5*exp(-0.048*1.5) + 102.5*exp(-2R) = 100

Solving this, we obtain = 4.953%. (Note that this calculation does not take day count conventions and holiday calendars into account. See Section 7.2.)

Example 7.2

"Suppose that the 6-month, 12-month, 18-month, and 24-month OIS zero rates (with continuous compounding) are 3.8%, 4.3%, 4.6%, and 4.75%, respectively. Suppose further that the six-month LIBOR rate is 4% with with semiannual compounding. The forward LIBOR rate for the period between 6 and 12 months is 5% with semiannual compounding. The forward LIBOR rate for the period between 12 and 18 months is 5.5% with semiannual compounding. We show how the forward LIBOR rate for the 18- to 24-month period can be calculated.

Suppose the two-year swap rate is 5%. The value of a two-year swap where LIBOR is paid and 5% is received is therefore zero. We know that the swap can be valued by assuming that forward rates are realized. The value of the first payment in the swap, assuming a principal of 100 is

0.5*(0.04 - 0.05) * 100 *exp(-0.038*0.5) = -0.4906.

The value of the second payment is

0.5*(0.05 - 0.05) * 100 *exp(-0.043*1.0) = 0

The value of the third payment is

0.5*(0.055 - 0.05) * 100 *exp(-0.046*1.5) = 0.2333

The value of the third payments is -0.4906 + 0 + 0.2333 = -0.2573. Suppose that the (assumed unknown) forward rate for the final payment is F. For the swap to be worth zero, we must have

0.5 * (F - 0.05) * 100 * exp(-0.0475*2) = 0.2573"

 

[SP_SK]

hi David, thanks for the explanation and I do understand the reason why hull is using ois rather than libor. your two sentences in bold one in black "This 5% swap rate means that a bond with a principal of $100 and a semiannual coupon of 5% per annum sells for par." and the one in red "Suppose the two-year swap rate is 5%. The value of a two-year swap where LIBOR is paid and 5% is received is therefore zero." are causing some confusion for me. the first one I understand because a bond having the same rate as the coupon will be priced at par. but the second one where the value is said to be zero, can you explain why is that not valued different than 0 ? is it because the way the swaps will be priced at initiation to be equal to 0 ?

 

[David Harper CFA FRM]

Hi @SP_SK This can be very challenging but the way that I look at it is: the 2nd version (10th edition) is the more natural. I think it is more natural to start with that valuation: in this second version, we are given the discount rates (OIS rates; 3.8%, 4.3% ...) which will discount future net flows. Further, we are given the basis for the determination of the floating rate payments, which cannot be known beyond the first but are predicted by the LIBOR forward rates (LIBOR forward rates: 4%, 5%, 5.5%). Just as you suggest, we can solve for the final 2-year LIBOR forward rate because we assume that a 5.0% swap rate is fair deal with net present value equal to zero; that means pay fixed at 5.0% in exchange for receiving floating LIBOR, but the net cash flow is discounted at OIS. This is akin to valuation of the swap "as if FRAs," and it must be done this way because the basis for determining the floating rate is not the discount rate.

The first example is a "shortcut" which can be done because the floating rate basis is the same as the discount rate (i.e., LIBOR in both cases). Below, because we can use this is future revisions, I have "proved this" by showing the first example, Hull 7-1 in 9th edition. He shows the solution of the 2-year zero rate = 4.953% (my cell performs the exact solution; the XLS is here https://www.dropbox.com/s/xy3jqpzc1g098uo/083018-hull-ex-71-versus-72.xlsx?dl=0). The upper panel illustrates the shortcut which assumes the elegant idea that a floater prices to par.

But in the bottom panel takes this 9th edition Example 7-1 and re-casts it into the 10th edition Example 7-2. This shows that an alternative is to solve for the zero rate by assuming this swap has NPV = 0 per:

0.5*(4.04% - 5.0%) * 100 * exp(-0.04*0.5) + 0.5*(5.06% - 5.0%) * 100 * exp(-0.045*1.0) + 0.5*(5.47% - 5.0%) * 100 * exp(-0.048*1.5) + 0.5*(5.49% - 5.0%) * 100 * exp(-0.04953*2.0) = 0

.. notice I do need to convert continuous zero rates into semi-annual forward rates; e.g., spots of 4.5% continuously @ 1.0 year and 4.8% @ 1.5 years imply a semi-annual forward rate, F(1.0, 1.5) of ~5.47%, which is the basis of the floating leg.

See how that's identical to the approach in the 10th edition? It's a special case because there LIBOR is the floating rate index and the discount rate such that the "shortcut" is available. But the shortcut actually does assume NPV = 0, but it already knows the floater prices to 100, so it only needs to solve for the fixed-rate (as if bond) leg equal to 100 also. I hope that clarifies!

 

[SP_SK]

Hi David, I need help for swaps chapter 7 hull, to understand comparative advantage. the study notes, page 104 sys that BBB corp will pay AAA 4.350 and I cannot understand how this number is getting derived. can you please explain. I have understood the difference of differences concept but now having trouble for the next step.

 

[SP_SK]

Hi David, I need help for swaps chapter 7 hull, to understand comparative advantage. the study notes, page 104 sys that BBB corp will pay AAA 4.350 and I cannot understand how this number is getting derived. can you please explain. I have understood the difference of differences concept but now having trouble for the next step.

please ignore I have been able to solve this thanks.