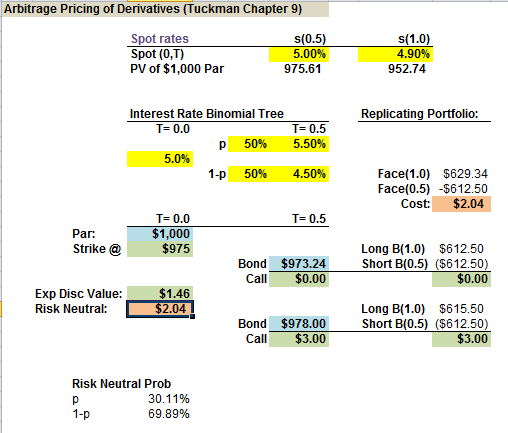
In the chapter, it always assumes that the future spot rate is going to go up. All of the RN probs of going up are higher than the ones going down (positive drift). This, in turn makes the option less valuable than its expected value. If, however, the spot rates were favored to go down (negative drift), wouldnt this make the price of the option greater than the expected value (because we would be discounting at a lower rate than the average of the two nodes)?
Thanks!
Shannon
Thanks!
Shannon