P.2 T5 Dowd study notes pg 10 confidence intervals
- Thread starter Stella G
- Start date
-
- Tags
- confidence-intervals dowd
Hi @Stella.gkotsi Good question, because our current note really does not explain this adequately, sorry (cc: @Nicole Seaman including another edit for this note). So it is implementing Dowd's 3.27 ....
.... he shows the calculation. Although mine is generated in its own excel (see sheet here https://www.dropbox.com/s/cpxnh4ituxnvpd9/0204-dowd-3-27.xlsx?dl=0), as below. For Bin Width = 0.10 (ie, first column):
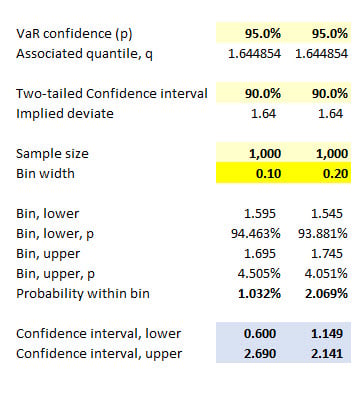
.... he shows the calculation. Although mine is generated in its own excel (see sheet here https://www.dropbox.com/s/cpxnh4ituxnvpd9/0204-dowd-3-27.xlsx?dl=0), as below. For Bin Width = 0.10 (ie, first column):
- f(q) = "Probability within bin" = 1 - 94.463% - 4.505% = 1.032%
- Per formula 3.27, Lower CI = 1.645 - 1.645*sqrt[4.505%*(1-4.505%)/1000]/1.032% = 0.600, and
- Upper CI = 1.645 + 1.645*sqrt[4.505%*(1-4.505%)/1000]/1.032% = 2.690. I hope that's helpful!
Thanks a lot @David Harper CFA FRM - that’s very helpful.
Hi @anniedu Dowd shows the derivation (see below) of the standard error of the quantile estimator on page 70:
Source -- Measuring Market Risk, 2nd Edition, Kevin Dowd
Source -- Measuring Market Risk, 2nd Edition, Kevin Dowd
Hi @David Harper CFA FRM
Thank you for the example, I was wondering why p is equal to 4.505% in the example for the calculation of confidence interval.
Thank you for the example, I was wondering why p is equal to 4.505% in the example for the calculation of confidence interval.
Hi @txiong It's a function of the selected bin width, h, about which Dowd says (emphasis mine) "in addition, the quantile standard error depends on the probability density function f (.) – so the choice of density function can make a difference to our estimates – and also on the bin width h, which is essentially arbitrary." (Dowd MMR p 70).
My left column above recreates Dowd's Example 3.7 which assumes bin width h = 0.10 but leads to wide CIs. Says Dowd, "The confidence interval narrows if we take a wider bin width, so suppose that we now repeat the exercise using a bin width h = 0.2, which is probably as wide as we can reasonably go with these data. q now falls into the range 1.645 ± 0.2/2 = [1.545, 1.745]."
... so my right column reproduces that; eg., 1.645 + 0.2/2 = 1.745. Then =1-NORM.S.DIST(1.745,TRUE) = 4.051%. Just like in the first column, =1-NORM.S.DIST(1.695,TRUE) = 1 - N(1.695) = 4.505%, where 1.695 = N^(-1)(95.0%) + 0.10/2 where 0.10 is the bin width. I hope that's helpful,
My left column above recreates Dowd's Example 3.7 which assumes bin width h = 0.10 but leads to wide CIs. Says Dowd, "The confidence interval narrows if we take a wider bin width, so suppose that we now repeat the exercise using a bin width h = 0.2, which is probably as wide as we can reasonably go with these data. q now falls into the range 1.645 ± 0.2/2 = [1.545, 1.745]."
... so my right column reproduces that; eg., 1.645 + 0.2/2 = 1.745. Then =1-NORM.S.DIST(1.745,TRUE) = 4.051%. Just like in the first column, =1-NORM.S.DIST(1.695,TRUE) = 1 - N(1.695) = 4.505%, where 1.695 = N^(-1)(95.0%) + 0.10/2 where 0.10 is the bin width. I hope that's helpful,
Hi @David Harper CFA FRM , Thank you for the detail explanation. I should be more specific about my question. I’m sorry if this is a stupid question but I was wondering why we always use the upper part of the bin.
Hi @txiong We don't, I was merely illustrating with the upper bound, because the lower bound has the same margin of error. What is the point of this exercise? We have estimated a VaR, which is just a quantile of the distribution; in the case of the standard normal, N(0,1), the 95.0% VaR is 1.645 because 5.0% of the standard normal's area is greater than 1.645. However, we recognize the 1.645 is only an estimate, so this is an estimate to carve a confidence interval around the estimate! The confidence interval looks like:
1.645 +/- z(α)*se(q), where z(α) is the deviate based on confidence, and se(q) is the standard error.
In the right-column above, this CI is:
Lower bound: 1.645 - 1.645 * 0.301375 = 1.149
Upper bound: 1.645 + 1.645 * 0.301375 = 2.141
See how that is a 90.0% CI = {1.149, 2.141}, around 1.645. We could carve instead a 99.0% CI:
Lower bound: 1.645 - 2.58 * 0.301375 = 0.869
Upper bound: 1.645 + 2.58 * 0.301375 = 2.421, and this is a 99.0% CI= {0.869, 2.421}, around 1.645
but the se(q) of 0.301375 did not change. It is given by sqrt[p*(1-p)/n]/f(q) = sqrt[4.051%*(1-4.051%)/1,000]/[1-93.881% - 4.051%] = 0.301374972. Both upper/lower bins are used, as Dowd explains (emphasis mine) "The confidence interval narrows if we take a wider bin width, so suppose that we now repeat the exercise using a bin width h = 0.2, which is probably as wide as we can reasonably go with these data. q now falls into the range 1.645 ± 0.2/2 = [1.545, 1.745]. p, the probability of a loss exceeding 1.745, is 0.0405, and the probability of profit or a loss less than 1.545 is 0.9388." Thanks,
1.645 +/- z(α)*se(q), where z(α) is the deviate based on confidence, and se(q) is the standard error.
In the right-column above, this CI is:
Lower bound: 1.645 - 1.645 * 0.301375 = 1.149
Upper bound: 1.645 + 1.645 * 0.301375 = 2.141
See how that is a 90.0% CI = {1.149, 2.141}, around 1.645. We could carve instead a 99.0% CI:
Lower bound: 1.645 - 2.58 * 0.301375 = 0.869
Upper bound: 1.645 + 2.58 * 0.301375 = 2.421, and this is a 99.0% CI= {0.869, 2.421}, around 1.645
but the se(q) of 0.301375 did not change. It is given by sqrt[p*(1-p)/n]/f(q) = sqrt[4.051%*(1-4.051%)/1,000]/[1-93.881% - 4.051%] = 0.301374972. Both upper/lower bins are used, as Dowd explains (emphasis mine) "The confidence interval narrows if we take a wider bin width, so suppose that we now repeat the exercise using a bin width h = 0.2, which is probably as wide as we can reasonably go with these data. q now falls into the range 1.645 ± 0.2/2 = [1.545, 1.745]. p, the probability of a loss exceeding 1.745, is 0.0405, and the probability of profit or a loss less than 1.545 is 0.9388." Thanks,
Hi @David Harper CFA FRM, this is much clear for me now. Thank you!
bollengc
Member
hi @David Harper CFA FRM and @Nicole Seaman,
I am now back for FRM part 2 and started with T5-R1.
I had troubles understanding as well the example and Dowd formula 3.27 (page 12 of T5-R1-P2-Dowd-v21) but found that thread that contains all explanation! (thanks, super clear)
The video also mentions the learning spreadsheet but the computation part cannot be found in the learning spreadsheet located in the study planner.
My suggestion would be to maybe add a tab in the learning spreadsheet of the study planner for that chapter and/or maybe add the link to this forum topic in the study notes (footnote link?)?
have a nice day
Camille
I am now back for FRM part 2 and started with T5-R1.
I had troubles understanding as well the example and Dowd formula 3.27 (page 12 of T5-R1-P2-Dowd-v21) but found that thread that contains all explanation! (thanks, super clear)
The video also mentions the learning spreadsheet but the computation part cannot be found in the learning spreadsheet located in the study planner.
My suggestion would be to maybe add a tab in the learning spreadsheet of the study planner for that chapter and/or maybe add the link to this forum topic in the study notes (footnote link?)?
have a nice day
Camille
Hi @bollengc Yes, I've included your recommendation in our PM (see below). Thank you for taking the time to offer such a specific recommendation!
Similar threads
