[email protected]
Active Member
Hi David,
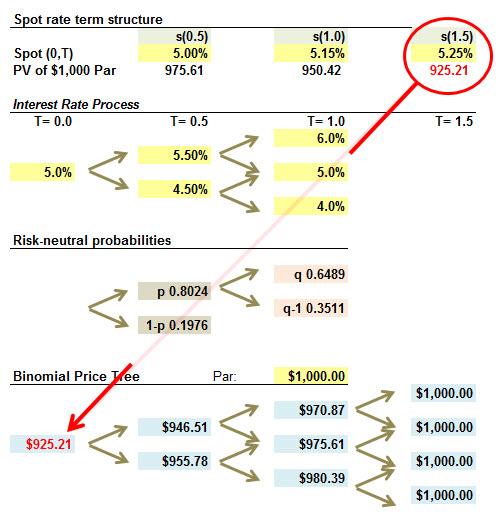
In the study notes and spreadsheet pack, I am struggling to understand how prices were calculated for the 3 step binomial (tab 29,7) for 946,51 and 955,78.
I get how to calculate (q) and (q-1), but this requires us to know 946,51 and 955,78 values from the earlier period.
But using the risk-averse values of (p) and (p-1) i.e. 80%/20%, I am struggling to work out the 946,51 and 955,78 and how they can be derived from the 925,21 T0 value.
Can you help?
Thanks
In the study notes and spreadsheet pack, I am struggling to understand how prices were calculated for the 3 step binomial (tab 29,7) for 946,51 and 955,78.
I get how to calculate (q) and (q-1), but this requires us to know 946,51 and 955,78 values from the earlier period.
But using the risk-averse values of (p) and (p-1) i.e. 80%/20%, I am struggling to work out the 946,51 and 955,78 and how they can be derived from the 925,21 T0 value.
Can you help?
Thanks